How does factoring help in solving the polynomials?

Factoring of the polynomial is the reverse method of the multiplication of the polynomial. Consider a polynomial axn + bxn-1 +kcxn-2 + ….+kx+ l, where each variable “x” is accompanied by a constant, and these coefficients are called the polynomial of the degree of “n”. The factoring calculator helps to solve the variable of degree “n” in various forms, like in monomials, binomials, trinomials, and so on.

The polynomials are separated by the addition or subtraction sign. The factoring finder calculator helps to make various combinations of the polynomials. The polynomial factoring calculator can make various combinations to find the solution to the question.

Example:

Sometimes we need to add two factors here we need to add a factor

x2+5x+6=0

x2+3x+2x+6=0

Then

x(x+3)+2(x+3)=0

(x+2)(x+3)=0

x+2=0, x+3=0

x=-2, x=-3

What is the process of factorization?

The process of factoring a given polynomial or mathematical expression is known to be factorization. Factors are the integers that are multiplied to find the original values. To find the factors of binomial we normally use a factoring calculator. If an expression is trinomial then we use the trinomial calculator. 

For example, consider the factors of number 18 are 2, 3, 6, 9, and 18, Now we can make various combinations of 18. This combination can be written as:

18 = 2 x 9

18 = 2 x 3 x 3

18 = 3 x 6

How do we factorize the polynomials?

When we factorize the polynomial, we multiply the factors by the factoring calculator to find the original factor. Now consider the factors of x2+5x+6 are (x + 2) (x + 3). Here when we are multiplying  (x + 2) (x + 3), the original factor is produced which is x2+5x+6. Now after completing the factorization process the factors are x=-2 and the x = -3

Example: 

Factorize x2-15x+50

Find and produce two numbers when added generate -15 and when multiplied give +50.

When we are using the factoring calculator, the factors are produced automatically, here we are doing the whole procedure.

When -5 and -10 are two numbers, when added produce “-15” and when multiplied produce “50”.This can be explained by the following explanation.

Then

(-5) + (-10) = -15, as (-)+(-)= –

(-5) x (-10) = 50  as (-) x (-)= +

The given polynomial can be written as;

x2-5x-10x+50=0

x(x-5)-10(x-5)=0

The 

(x-5)(x-10)=0

Hence the factors calculated by the factoring calculator are

 (x – 5) and (x – 10).

Factoring Using Identities

The factorization of the polynomial can also be done by using algebraic identities. The most common identities used by the factoring calculator in terms of factorization are:

• (a + b)2 = a2 + 2ab + b2
• (a – b)2 = a2 – 2ab + b2
• a2 – b2= (a + b)(a – b)

The factoring calculator can identify the identity and generate the result automatically.

Example:

Factorize (x2 – 112)

a2 – b2= (a + b)(a – b), the identity is used as 

(x2 – 112) = (x)^2-(11)^2 = (x+11) (x-11)

So the above polynomial as;

(x+11) (x-11)

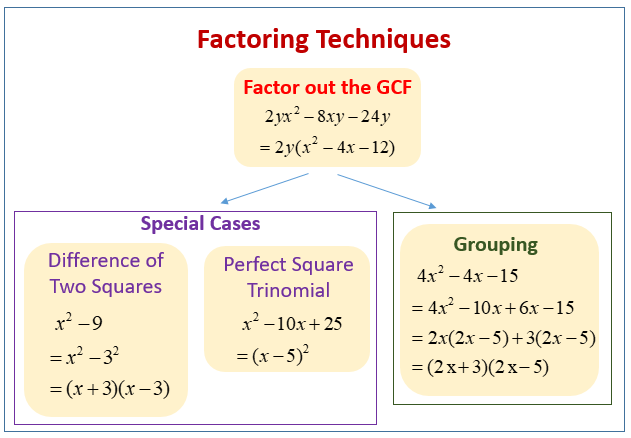
Types of Factoring?

The  types of factoring may include various methods the common types of the factorization of the polynomial include :

1. Finding the GCF or Greatest Common Factor.
2. Factorization by Grouping
3. Finding the Difference in Two Squares.
4. Sum or Difference in Two Cubes.
5. Factor the Trinomial or trinomial method.

Conclusion:

The factoring calculator utilizes all the various types of factorization to solve various polynomials. For simplicity, it is better to identify the factors of various numbers and the polynomials.